DEM11 Lissajous Figures

Objective:

– To demonstrate Lissajous Figures.

Apparatus:

• Oscilloscope – Tektronix TDS 2012C
• 2 – BK Precision 4011A 5MHz Function Generators

Jules Antoine Lissajous (March 4, 1822 – June 24, 1880) was a French mathematician, after whom Lissajous figures are named. Among other innovations, Lissajous invented the Lissajous apparatus, a device that creates the figures that bear his name. In it, a beam of light is bounced off a mirror attached to a vibrating tuning fork, and then reflected off a second mirror attached to a perpendicularly oriented vibrating tuning fork (usually of a different pitch, creating a specific harmonic interval), onto a wall, resulting in a Lissajous figure.

A Lissajous figure is produced on an oscilloscope by taking two sine waves and displaying them at right angles to each other in the XY mode. In the following examples the two sine waves have equal amplitudes.

Lissajous figures often appeared as props in science fiction movies made during the 1950’s. One of the best examples can be found in the opening sequence of The Outer Limits TV series.

(“Do not attempt to adjust your picture–we are controlling the transmission.”) The pattern of crisscross lines is actually a Lissajous figure.

Note:

It is easier to synchronize both sine waves when the waveform display is in YT mode. After matching both frequency and magnitude of the sine waves (both waves are overlapping each other), change waveform display to XY mode.

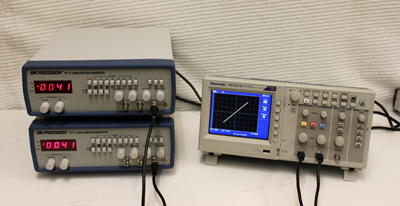
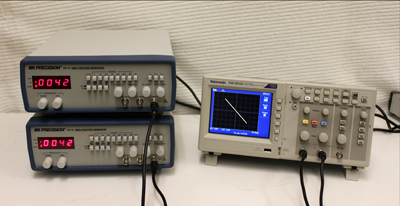
When the two sine waves are of equal frequency and in-phase, you get a diagonal line to the right.	When the two sine waves are of equal frequency and 180 degrees out-of-phase, you get a diagonal line to the left.
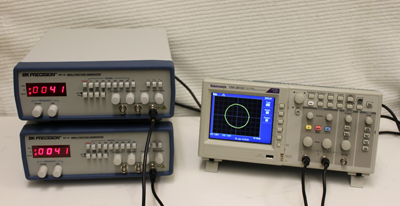
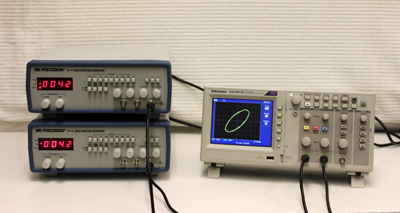
When the two sine waves are of equal frequency and 90 degrees out-of-phase, you get a circle.	In this picture both input frequencies are identical, but the phase variance between them creates the shape of an ellipse
If the two sine waves are in phase but the frequency of the horizontal sine wave is twice the frequency of the vertical sine wave you get the pattern shown here.	This shows the sine wave 90 degrees out-of-phase with the frequency of the horizontal sine wave three times the frequency of the vertical sine wave.